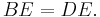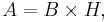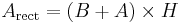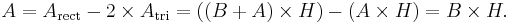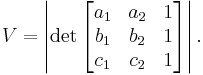Parallelogram
| Parallelogram | |
|---|---|
 This parallelogram is a rhomboid as its angles are oblique. |
|
| Type | Quadrilateral |
| Edges and vertices | 4 |
| Symmetry group | C2 (2) |
In geometry, a parallelogram is a quadrilateral with two pairs of parallel sides. In Euclidean Geometry, the opposite or facing sides of a parallelogram are of equal length and the opposite angles of a parallelogram are of equal measure. The congruence of opposite sides and opposite angles is a direct consequence of the Euclidean Parallel Postulate and neither condition can be proven without appealing to the Euclidean Parallel Postulate or one of its equivalent formulations. The three-dimensional counterpart of a parallelogram is a parallelepiped.
The etymology (in Greek παραλληλ-όγραμμον, a shape "of parallel lines") reflects the definition.
Contents |
Properties
- Opposite sides of a parallelogram are equal in length.
- Opposite angles of a parallelogram are equal in measure.
- The area,
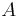 , of a parallelogram is
, of a parallelogram is 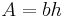 , where
, where 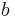 is the base of the parallelogram and
is the base of the parallelogram and  is its height.
is its height. - Opposite sides of a parallelogram will never intersect.
- The area of a parallelogram is twice the area of a triangle created by one of its diagonals.
- The area of a parallelogram is also equal to the magnitude of the vector cross product of two adjacent sides.
- The diagonals of a parallelogram bisect each other.
- Any non-degenerate affine transformation takes a parallelogram to another parallelogram.
There is an infinite number of affine transformations which take any given parallelogram to a square. - A parallelogram has rotational symmetry of order 2 (through 180°). If it also has two lines of reflectional symmetry then it must be a rhombus or a rectangle.
- The perimeter of a parallelogram is 2(a + b) where a and b are the lengths of adjacent sides.
Types of parallelogram
- Rhomboid - A quadrilateral whose opposite sides are parallel and adjacent sides are unequal, and whose angles are not right angles
- Rectangle - A parallelogram with four angles of equal size (right angles).
- Rhombus - A parallelogram with four sides of equal length.
- Square - A parallelogram with four sides of equal length and four angles of equal size (right angles).
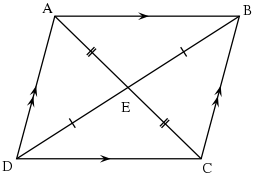
Proof that diagonals bisect each other

To prove that the diagonals of a parallelogram bisect each other, we will use congruent triangles:
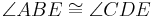 (alternate interior angles are equal in measure)
(alternate interior angles are equal in measure)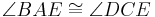 (alternate interior angles are equal in measure).
(alternate interior angles are equal in measure).
(since these are angles that a transversal makes with parallel lines 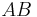 and
and 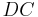 ).
).
Also, side AB is equal in length to side DC, since opposite sides of a parallelogram are equal in length.
Therefore triangles ABE and CDE are congruent (ASA postulate, two corresponding angles and the included side).
Therefore,
Since the diagonals  and
and 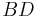 divide each other into segments of equal length, the diagonals bisect each other.
divide each other into segments of equal length, the diagonals bisect each other.
Separately, since the diagonals  and
and 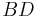 bisect each other at point
bisect each other at point 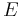 , point
, point 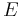 is the midpoint of each diagonal.
is the midpoint of each diagonal.
The area formula

The area formula,
can be derived as follows:
The area of the parallelogram to the right (the blue area) is the total area of the rectangle less the area of the two orange triangles. The area of the rectangle is
and the area of a single orange triangle is
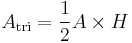 or
or 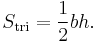
Therefore, the area of the parallelogram is
Computing the area of a parallelogram
Let 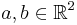 and let
and let ![V=[a\ b]\in\R^{2\times2}](/I/d4a4d61871d9369ef09c27599408398c.png) denote the matrix with columns
denote the matrix with columns 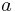 and
and 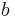 . Then the area of the parallelogram generated by
. Then the area of the parallelogram generated by 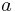 and
and 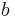 is equal to
is equal to 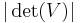
Let 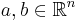 and let
and let ![V=[a\ b]\in\R^{n\times2}](/I/7ee63da52bc6cdd0d3bb9d38d1870354.png) Then the area of the parallelogram generated by
Then the area of the parallelogram generated by 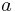 and
and 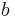 is equal to
is equal to 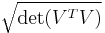
Let 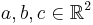 . Then the area of the parallelogram with vertices at a, b and c is equivalent to the absolute value of the determinant of a matrix built using a, b and c as rows with the last column padded using ones as follows:
. Then the area of the parallelogram with vertices at a, b and c is equivalent to the absolute value of the determinant of a matrix built using a, b and c as rows with the last column padded using ones as follows:
See also
- Fundamental parallelogram
External links
- Parallelogram and Rhombus - Animated course (Construction, Circumference, Area)
- Weisstein, Eric W., "Parallelogram" from MathWorld.
- Interactive Parallelogram --sides, angles and slope
- Area of Parallelogram at cut-the-knot
- Equilateral Triangles On Sides of a Parallelogram at cut-the-knot
- Definition and properties of a parallelogram with animated applet
- Interactive applet showing parallelogram area calculation interactive applet